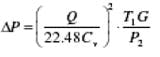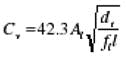Web Exclusive — Whole-sizing pneumatic motion systems
By Curtis Harvey,
Foth Production Solutions LLC, Lake Elmo, Minn.
Too often, flow coefficient, CV, is used to size a valve without giving consideration to the other components in a pneumatic circuit. In the past, a focus on the valve alone often sufficed because the cost of air was considered “free” and designers tended to significantly oversize the valve. Today’s emphasis on better energy management and optimized performance requires a more-exacting method to properly size pneumatic motion systems.
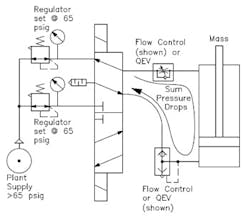
As shown in this pneumatic circuit, designers must account for pressure drop across the valves, but also for other system components such as fittings, flow controls, and tubing.
Using the following design process, sustainability conscious users will increase their “green footprint” by decreasing their air and energy usage with a corresponding drop in CO2 emissions. Reductions are realized by using smaller, fewer, and less expensive components. Debugging and rework time is minimized and the entire system runs more smoothly and efficiently. Finally, suggestions are offered to better optimize circuit designs.
Predicting flow in pneumatic systems
The CV equation:
provides a standardized means of predicting flow through a valve or other systems component. However, because the valve is only one part of a complete system, every component that potentially causes a flow restriction or pressure drop must be considered as part of the overall design.
Additional factors to consider:
- A programmable logic controller (PLC) with a “scan time” typically controls the valve. Scan time is the amount of time the processor takes to evaluate and execute a set of instructions before repeating the entire process.
- Consider the response time for the valve’s internal mechanism to switch from fully closed to fully open once it receives a signal from the PLC.
- Air flow is restricted due to the inside diameter of cylinder and valve port fittings.
- Flow characteristics of the air lines between a valve and cylinder differ depending on their material and whether they are rigid or flexible.
- Air-lines add volume to the system that must be charged and discharged every cycle.
- Additional pressure losses and delays are caused by fittings that are not straight.
- Flow controls restrict airflow even in their full-open position.
- The load cannot move until a sufficient pressure difference develops across the cylinder piston to overcome gravity and friction caused by seals and guide bearings.
Practical considerations
To put these factors into practical terms, let’s look at the process involved in designing a typical system. Consider a pneumatic motion system initially consisting of:
- A pneumatic air cylinder, rod up, pushing a 10-lb mass vertically 3 in. in 0.170 sec.
- A non-lubricated supply pressure of 79.70 psia (65 psig) and an exhaust pressure of 14.70 psia.
- A PLC with a scan time of 0.010 sec.
- A valve shifting from closed to open in 0.032 sec for a valve with CV=1.
- Prior to the valve shifting, a 79.70 psia pressure is holding the load down with the bottom of the piston fully exhausted. This pressure can differ from the supply pressure that lifts the cylinder.
- 14-in. lengths of flexible tubing with no angled fittings connect the valve and cylinder.
- No external friction sources exist.
- No flow controls will be used.
Let’s also make these basic observations and assumptions:
1. The CV equation above applies equally well to all circuit components, not just the valve.
2. Flows are not the same for all components in a circuit branch. The cylinder fitting ‘sees’ the air entering/exiting the cylinder, while the valve fitting ‘sees’ the air of both the cylinder and the air line entering/exiting through the valve.
3. Cylinder motion is “bang-bang” and can be represented by a constant acceleration where velocity increases linearly. The cylinder provides the acceleration force but external geometry stops the load abruptly without cushions or shock absorbers. This is used to calculate an average force the cylinder must generate. Actual motion forces and velocities will change significantly but the work done (effort X displacement) does not change much and does not particularly depend on the motion profile.
4. The effects of temperature change and air leakage are negligible.
5. There are negligible differences between static and dynamic friction.
System sizing
The following circuit calculations are iterative in nature so a software program such as Excel or MathCAD is recommended. Here are the key considerations.
Physics: First, calculate the required cylinder force. The maximum time that the cylinder “sees” air is 0.170 sec, less the PLC scan time, and less half the valve solenoid shift time. That leaves 0.144 sec for the actual move time plus the delay time for sufficient pressure to build up before the load moves. Start with a “guess” move time of about two-thirds of 0.144 sec, or in this case about 0.096 sec. Average velocity is 3 in./0.096 sec, or 31.25 in/sec.
Assuming constant acceleration, peak velocity is twice this or 62.5 ips. Divide peak velocity by 0.096 sec. to get acceleration, 651 ips2 or 1.685 G. Thus, the required lifting force is 1.685 X 10 lb (due to acceleration) + 10 lb (due to gravity) = 26.85 lb force. If there are other forces, such as guide friction, they would be added here.
Cylinder: The cylinder should be sized to provide an extra 33% to 100% of the calculated required force. This is necessary to overcome the pressure losses from the other components in the circuit. In this case, design for a cylinder force of between 36 lb and 54 lb at 65 psig.
Because Force = Pressure x Area, the bore should be between 0.84 in. and 1.03 in. with no rod. We’ll start with an oversize 1.50 in. diameter cylinder with a 0.625 in. diameter rod and 1/8 in. NPT ports. From the manufacturer’s catalog, friction is 13 psi. This is the minimum pressure needed to overcome the cylinder’s internal friction and move the piston with no load. A starting guess of ¼-in.-ID flexible tubing is made to supply air to the cylinder.
Method: We’ll sum all the pressure drops around the circuit, starting at the valve exhaust port (atmospheric pressure) and ending at the valve supply port (regulated supply pressure). These pressure drops should add up to the pressure difference between the supply pressure and atmospheric pressure. This is very similar to Kirchhoff’s Voltage Law used by electrical engineers to solve electrical circuits.
Valve exhaust port: To size the valve, first calculate flow during motion through the valve exhaust port. Calculate the volume being evacuated, divide by time, and then multiply by the pressure ratio to convert to standard conditions.
Air-cylinder exhaust volume = (3)(π/4)(1.52 - 0.6252) = 4.38 in3.
Air-line exhaust volume = (14)( π/4)(0.252)(79.7-14.7)/79.7 = 0.56 in3.
The air line does not get completely exhausted; one atmosphere air pressure remains. Total exhaust volume = 4.94 in3.
Divide this by 0.096 sec to get 51.5 in3/sec, or 1.787 cfm. This is at full pressure.
Multiply by 79.7/14.7 (pressure ratio) to convert to standard conditions, 9.69-scfm exhaust.
In the same manner, the supply flow during motion (without the rod) through the valve supply port is 11.50-scfm supply.
An assumption is necessary at this point. Valve manufacturers recommend designing for a 2- to 10-psi pressure drop across the valve exhaust port. We’ll assume a 6-psi drop.
Substituting Q = 9.69, P = 6, and P = 14.7 into the CV equation, recommended valve CV = 1.055.
Choose a standard valve with CV = 1.0 with ¼-in. NPT ports. Because flow requirements are unchanged, calculate pressure drop based on the smaller valve. Rearranging the CV equation,
valve exhaust-port pressure drop P = 6.67 psi, well within the 2- to 10-psi requirement.
There is a critical pressure ratio for each circuit component, P2/P1, that must be greater than or equal to 0.528. If P2/P1 equals 0.528, the air flow is sonic or ‘choked’ and is at the maximum flow possible. If P2/P1 is less than this value, flow is impossibly high and must be reduced until P2/P1 is equal to this value. For this component P2/P1 = 14.7/21.37 = 0.688, so it’s subsonic and OK.
Exhaust air line: Determine air line CV using
The line-friction coefficient fl for flexible tubing is 0.02 (use 0.03 for rigid steel pipe). This yields an exhaust air line CV = 1.962. Note that if the line has 90° fittings, add 48ndf to the length of the line before calculating air-line CV. The flow used here is based on the cylinder volume plus the air line volume or 9.14 scfm. Using 1.962 CV, a downstream pressure of 23.68 psi (14.7 + 6.67 + 2.30) and 9.14 scfm from the CV equation, exhaust air-line pressure drop = 0.96 psi. P2/P1 = 23.68/(23.68+0.96)=0.961, so it’s OK.
Exhaust and supply flow controls: If you don’t have an actual CV from the manufacturer, calculate CV for flow controls with the fitting equation, but use the air-lines inside diameter. If a flow control were used here with a 0.25-in.-diameter line, CV would be minimum 1.125 (full open). Since flow controls are typically in the cylinder port, the flow used to calculate P would be based on the cylinder volume only or 8.59 scfm. It is not in the circuit so we’ll move on to the next component.
Exhaust cylinder fitting: A 1/8-in. NPT straight fitting has a 0.19-in. ID. Using the fitting CV equation, exhaust cylinder fitting CV = 0.650.
This gives a downstream pressure of 24.64 psi (23.68 + .96) and 8.59 scfm.
From the CV equation, exhaust cylinder fitting pressure drop = 7.41 psi. P2/P1 = 24.64/32.05=0.769, so it’s OK.
Cylinder: At this point, piston exhaust side pressure = 32.05 psia (24.64 + 7.41). Add to this the cylinders 13-psi internal friction to get 45.05 psia. Multiply this by the area to get a resistive force of 65.79 lb. Add the required cylinder output force of 26.85 lb to get a supply-side piston force requirement of 92.64 lb. Divide this by the area of a 1.50-in. diameter piston with no rod and you’ll get a supply side pressure = 52.42 psia.
Cylinder-supply fitting: Using CV = 0.650 for 1/8-in. NPT, a downstream pressure of 52.42 psia and 10.40 scfm, cylinder supply fitting pressure drop is 5.10 psi. P2/P1 = 52.42/57.52=0.911, so it’s OK.
Supply air line: CV = 1.962, downstream pressure = 57.52 psia, Q = 10.95 scfm, and supply air-line pressure drop = 0.57 psi. P2/P1 = 57.52/58.09=0.990, so it’s OK.
Supply-valve fitting: CV = 1.411, downstream pressure = 58.09 psia, Q = 11.50 scfm, and supply valve fitting pressure drop = 1.19 psi. P2/P1 = 58.09/59.28=0.980, so it’s OK.
Valve-supply port: CV = 1.0, downstream pressure = 59.28 psia, Q = 11.50 scfm, and valve supply port pressure drop = 2.33 psi. P2/P1 = 59.28/61.61=0.962, so it’s OK. This adds up to a final supply pressure = 61.61 psia, with 18.09 psi to spare.
Delay time: When the valve shifts from closed to open, the pressure previously built up in the cylinder (rod end) begins to bleed out the high-volume exhaust end while, simultaneously, pressure builds on the low-volume supply end. Because cylinder volume on the supply end is very small, supply pressure builds quickly. The time delay before motion begins is mostly due to the time it takes for the exhaust pressure to fall below the supply pressure and develop the necessary pressure difference across the piston to overcome gravity and friction, and initiate motion.
Calculate delay time, td, as follows:
Td = PegVeks/qmvs2. Here, Pe = 79.70 – 32.05 psia (the piston low side pressure). Density of air is approximately 0.075 lb/ft3 at 528°R. Velocity of sound vs is approximately 1127 fps at 528°R. Ratio of specific heats ks for air = 1.4.
Therefore, Td = 0.059 sec. So the initial time estimate = 0.096 shift time + 0.059 delay time + 0.032/2 valve solenoid time + 0.010 PLC scan time = 0.181 sec.
Limitations: Besides the critical pressure ratio already discussed, it is recommended that no component in a circuit should exceed 15% of the absolute upstream pressure or 11.96 psi for this example. There was extra pressure to spare when the last pressure (79.70 - 61.61 psi) was calculated, and no pressure drop exceeded 11.96 psi. This indicates that the initial move-time guess can be improved and all the above calculations repeated until the last pressure equals the supply, one component is at the maximum pressure drop, or the pressure drop across the valve exhaust port exceeds 10 psi.
Results: Optimizing the shift time yields 0.146 sec (0.078 motion time + 0.042 delay time + 0.016 + 0.010) with a limiting maximum pressure drop of 10 psi across the valve exhaust port. Increasing the supply pressure will have little effect on speed and will increase delay time before motion begins.
Reality check: This pneumatic-motion circuit was set up using all the variables identified for the 1.50 in. diameter cylinder, except without a PLC. Without the PLC’s 0.01 sec delay, cylinder actuation time was 0.134 sec. That is approximately 0.002 sec faster (0.136 calculated without a PLC) than the initial estimate. The time for the actual cylinder to lower was 0.144 sec with no PLC, vs. a calculated time of 0.158 sec. Thus, there is good agreement between theory and actual conditions.
Economics plus: Assuming this cylinder operates 24/7, extending and retracting once every 3 sec, its operating cost will run about $182 per year based on air costing $0.50 per 1000 standard cubic feet. The energy consumed to create the compressed air produces about 620 lbs per year of CO2 based on 1.7 lbs of CO2 produced per 1000 standard cubic feet of air (gas ~ 1.3/1000, oil ~ 1.7/1000, or coal ~ 2.1/1000).
Reducing the 1.50 in bore cylinders raising and lowering pressures 5.5 psi to 74.2 psia (59.5 psig) improves its’ raise time from 0.146 sec. to 0.138 sec. and the lower time from 0.168 sec. to 0.157 sec. and saves about 7% in air and CO2. Reducing the supply pressure further provides additional savings while staying within our 0.170 sec. budget. Further gains are possible utilizing this same analysis method with dual air pressures and quick exhaust valves. See the optimized circuit table.
Selecting smaller cylinders, a 1.0625 in. diameter bore or a 0.875 in. diameter bore with smaller rod diameters will also work. The other component CVs can be reduced to provide additional savings. See the pneumatic circuit table.
|
Pneumatic circuit table |
|||||
|
System parameters |
1-1/2 diameter first pass |
1-1/2 diameter final pass |
1-1/2 diameter better time |
1-1/16 diameter cylinder |
7/8 diameter cylinder |
|
Cylinder diameter, in. |
1.500 |
1.500 |
1.500 |
1.063 |
0.875 |
|
Rod diameter, in. |
0.625 |
0.625 |
0.625 |
0.500 |
0.250 |
|
Exhaust pressure, psia |
14.70 |
14.70 |
14.70 |
14.70 |
14.70 |
|
Supply pressure, psia |
79.70 |
79.70 |
74.20 |
79.70 |
79.70 |
|
Hold down pressure, psia |
79.70 |
79.70 |
74.20 |
79.70 |
79.70 |
|
PLC scan time, sec |
0.010 |
0.010 |
0.010 |
0.010 |
0.010 |
|
Valve CV |
1.000 |
1.000 |
1.000 |
0.700 |
0.600 |
|
Valve port size, NPT |
1/4 |
1/4 |
1/4 |
1/4 |
1/8 |
|
Air-line length, in. |
14.00 |
14.00 |
14.00 |
14.00 |
14.00 |
|
Air-line ID, in. |
0.250 |
0.250 |
0.250 |
0.156 |
0.156 |
|
Valve-solenoid shift time, sec |
0.032 |
0.032 |
0.032 |
0.010 |
0.010 |
|
Target move time, sec |
0.170 |
0.170 |
0.170 |
0.170 |
0.170 |
|
Initial estimate, move+delay time, sec |
0.144 |
- |
- |
- |
- |
|
Move time, sec |
0.096 |
0.078 |
0.075 |
0.074 |
0.095 |
|
Air cylinder supply flow, scfm |
10.40 |
12.73 |
12.46 |
6.74 |
3.57 |
|
Air-line supply flow, scfm |
1.10 |
1.35 |
1.30 |
0.55 |
0.43 |
|
Total supply flow, scfm |
11.50 |
14.07 |
13.75 |
7.30 |
4.00 |
|
Air cylinder exhaust flow, scfm |
8.59 |
10.52 |
10.30 |
5.25 |
3.28 |
|
Air-line exhaust flow, scfm |
1.10 |
1.35 |
1.30 |
0.55 |
0.43 |
|
Total exhaust flow, scfm |
9.69 |
11.86 |
11.59 |
5.80 |
3.71 |
|
Peak velocity, in./sec |
62.50 |
76.52 |
80.45 |
80.78 |
63.05 |
|
Acceleration, G |
1.685 |
2.526 |
2.792 |
2.814 |
1.715 |
|
Pressure calculations |
|||||
|
Valve exhaust pressure, psi |
6.67 |
10.00 |
9.55 |
4.89 |
2.72 |
|
Exhaust valve-fitting pressure, psi |
2.30 |
2.99 |
2.91 |
0.90 |
1.96 |
|
Exhaust air-line pressure, psi |
0.96 |
1.23 |
1.20 |
4.24 |
1.79 |
|
Exhaust flow control pressure, psi |
0.00 |
0.00 |
0.00 |
0.00 |
0.00 |
|
Exhaust cylinder-fitting pressure, psi |
7.41 |
9.47 |
9.25 |
2.76 |
1.26 |
|
Supply cylinder-fitting pressure, psi |
5.10 |
6.42 |
6.07 |
1.51 |
0.41 |
|
Supply flow control pressure, psi |
0.00 |
0.00 |
0.00 |
0.00 |
0.00 |
|
Supply air-line pressure, psi |
0.57 |
0.71 |
.67 |
1.84 |
0.52 |
|
Supply valve-fitting pressure, psi |
1.19 |
1.49 |
1.42 |
0.36 |
0.50 |
|
Valve supply pressure, psi |
2.33 |
2.91 |
2.77 |
1.45 |
0.59 |
|
Results |
|||||
|
Maximum allowable pressure drop, psi |
11.96 |
11.96 |
11.13 |
11.96 |
11.96 |
|
Cylinder force output, lb |
26.85 |
35.26 |
37.92 |
38.14 |
27.15 |
|
Delay time, sec |
0.059 |
0.042 |
.038 |
0.050 |
0.070 |
|
Estimated total time, sec |
0.181 |
0.146 |
.138 |
0.139 |
0.180 |
|
Cost per year to operate |
$182.34 |
$182.34 |
$169.76 |
$86.87 |
$65.92 |
|
CO2 produced per year (lbs) |
620 |
620 |
577 |
295 |
224 |
|
Tabulated results are for the example circuit lifting a 10-lb mass 3 in. in 0.170 sec. or less. Cylinder ports are 1/8 NPT and there are no flow controls in the system. Note the 10 psi limiting P for the 1½-in. bore cylinder. |
|||||
This should be of considerable interest if you and/or your end users are trying to increase your “green” footprint or estimate the benefits from sustainability efforts. A 32% cost/CO2 reduction was obtained by adding a regulator to the 1.50-in. bore cylinder circuit. A 54% cost/CO2 reduction was obtained by adding regulators with quick exhaust valves (CV =0.72) to this same circuit. The 1½-in. bore cylinder provided a good example problem but should not be the final choice for this application. A 76% cost/CO2 reduction is possible using a 1-1/16-in. bore cylinder instead with regulators and quick exhaust valves. Further reducing the cylinder bore to 7/8-in. doesn’t appear to have as many advantages. There could be some small space savings and component cost reductions.
Non-recurring benefits include smaller, fewer, and cheaper components. Less CO2 is produced making smaller and fewer components. Space requirements are reduced. Cylinder stroke times are more predictable. Debug and assembly rework time is minimized. Machine delivery schedule has a better chance of being maintained or beat.
Recurring benefits include reductions in air usage and electric power consumption with the corresponding drop in CO2 production. Costs for providing and maintaining the air supply are reduced. Leakage due to lower pressures is reduced. Insights into pneumatic performance are gained by pneumatic designers as they adjust the parameters involved in sizing their circuit components.
Finally, I’ll leave you with one last thought. I’m told that CO2 is not the only byproduct of consuming energy that we should be concerned about here. There are more hazardous pollutants to consider such as carbon monoxide (CO), nitrogen oxides (NOX), sulfur oxides (SOX), fine particulates (PM10-particle matter under 10 micron size), and volatile organic compounds (VOCs). Today, limiting CO2 production to halt global warming seems to be on everyone’s radar, tomorrow. If we all start to think a little greener today, we should all breath a little cleaner tomorrow.
For more information, contact Curtis Harvey, lead mechanical engineer, Foth Production Solutions LLC, at [email protected] or visit www.foth.com.
Here are a few suggestions for optimizing your circuit designs:
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Symbols used and their meanings G = Specific gravity of air at one atmosphere, 68°F, and 36% relative humidity. Usually, G = 1. g = Acceleration of gravity ks = Specific-heat ratio; ks= 1.4 for air. n = Number of fittings Pe = Exhaust pressure, psi Ps = Supply pressure, psi P1 = Upstream pressure at T1, psia P2 = Downstream pressure, psia QEV=Quick Exhaust Valve qm = Mass flow rate ips=inches per second T1 = Upstream temperature, °R. Usually, T1= 528°R. Ve = Exhaust volume, in.3 |