This series of articles will describe a new non-linear model of a 4-way directional control valve. Model development for steady-state purposes proceeds in two main steps. The first step consists of the main, variable parts of the valve — for example, an idealized evaluation of the basic spool and sleeve assembly in a typical servovalve without regard to any subplate or manifold pressure losses. The second step includes the inevitable parasitic pressure losses in any practical implementation.
As such, the model is completely non-reliant on internal dimensions. Instead, it is based totally on measured, external performance data as collected in accordance with ISO 10770-1. The model applies equally to both servo and proportional valves.
Model input data must include:
• internal land-to-land leakage (work ports blocked) as a function of spool position and the pressure at which the leakage was measured. The land-toland component must be separated from pilot flow when data are collected according to ISO 10770-1,
• rated flow at full spool shift and the flow-rating pressure that was used in measuring the flow, and
• the target value of the overlap to be modeled, which can be any arbitrary amount of over or under lap and can be assigned differently to each of the four lands.
The completed model emulates the steady-state performance of the real source valve in the following ways:
• Simulated flow metering characteristics (looped work port flow) at any supply and tank pressure are a function of spool position for full shift in both directions.
• Simulated pressure metering characteristics (blocked work port pressure) at any pressure are a function of spool position for full shift in both directions.
• Valve over- and under-lap characteristics are based on the targeted input values, but require some trial and error to duplicate the precise value.
• Work port flows (under any work port pressure loading conditions and any supply and tank pressure) and overlap conditions can be balanced or unbalanced to any degree desired among the four lands so that manufacturing imperfections and mis-nulling of the valve can be emulated.
• Internal land-to-land leakage with work ports blocked at any supply and tank pressures can be represented.
Limitations and assumptions
The modeling procedure only applies to valves designed to produce linear work port flow metering characteristics, such as those from rectangular spool or sleeve metering slots. For simplicity, individual land leakages are assumed to be turbulent in over-lap regions of spool travel and are modeled to vary in inverse proportion to the amount of overlap at the instant spool position is governed by the Hagen Poiseuille equation. This equation calculates pressure drop of a fluid flowing through a long cylindrical pipe.
In all likelihood, internal land leakage is laminar in the large overlap spool positions. However, the amount of leakage through a blocked land is insignificant in most applications of servo and proportional valves.
The flow through any individual land, whether in a closed, open or transition (null zone) position, is assumed to vary with the square of the differential pressure drop across the land.
Spool position is the significant independent variable in the use of the model. Therefore, flow forces on the spool are not a part of the model. Flow forces must be modeled by analyzing forces acting on the spool and must be evaluated external to this valve model. This model predicts the above characteristics when the spool position is known.
Pilot flow (often given as a component of the internal leakage) is not included in this first step of the modeling procedure. Instead, it is evaluated in the second step, when parasitic losses are added to the model.
Given the above assumptions and limitations, a valve model emerges that allows for very simple analysis. But more importantly, the modeling process yields a set of closed equations that require no iterative methods to calculate the key parameters.
Model development
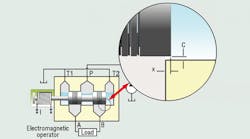
Four-way directional control valve modeling often begins with the analytical schematic showing the valve and its four variable metering lands in a bridge circuit configuration, Figure 1. The four variable orifices are all linked together mechanically because they are all assumed to be machined onto a single spool. The timing of the moving (spool) lands relative to the stationary (sleeve) lands is such that when the spool is shifted one way, KVPL,PA and KVPL,BT both open while KVPL,PB and KVPL,AT overlap (close).
Shifting the spool in the other direction causes the four lands to exchange their open-closed functions. This land timing results in a proportional and controllable flow in both amount and direction, giving full control of any load on the valve. Many of the details have been covered in previous editions “Motion Control.”
Valve construction
Although the modeling method to be covered shortly does not rely on any dimensions, some background on the construction and manufacturing of valves is helpful because they both affect performance curves. Also, the method of setting the parametric values for a given valve can be facilitated by viewing the parameter settings as similar to manufacturing the valve.
When discussing the performance of servo and proportional valves, a key characteristic is the amount of valve lap. Lap can be either under, over, or so-called zero lap, and lap refers to the amount that the spool must be shifted from center before the valve lands open. Underlap and overlap are generally unambiguous and can be recognized by a quick inspection of the flow metering characteristics for any particular valve. Quantifying them, however, is not standardized and not without its problems. Because no standard definitions exist, we’ll add the terms physical overlap and apparent overlap to the present discussion.
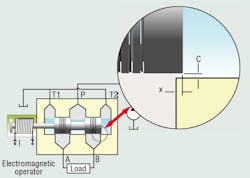
Physical overlap is the distance labeled X in Figure 2 whereas apparent overlap is lap based on how uniformly the flow metering passes through zero. The terms zero lap and valve opening are misleading because no universal definition exists for zero lap. Furthermore, a spool valve can never be closed.
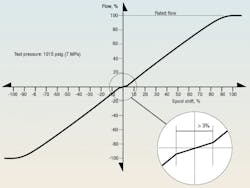
Consider zero lap. Figure 3 shows a flow metering graph for an ideal, zero lapped valve with its curve passing straight and perfectly through zero. It is often shown this way, but, alas, such a valve does not exist in reality. A real valve can be made to closely approximate this characteristic, but it is never strictly achieved. The reason is because the valve always has some radial clearance dimension (C in Figure 2) between the spool and its bore, or sleeve. So the closest approximation to Figure 3’s idealized, zero-crossing actually occurs when there is some degree of physical overlap. In other words, some overlap is required to obtain zero lap. This may sound contradictory, but only because of the term zero lap.
I prefer the term critical lap. Critical lap is a condition, not a dimension. It is defined as: a trial-and-error valve center lap condition wherein the flow metering curve passes through zero with the minimal change in the slope (flow gain), as idealized in Figure 3. The null zone is generally taken as that region near zero flow. In this discussion, a valve that has flow metering of Figure 3 would be better described as having apparent zero lap.
Consider the manufacturing process and what is meant by trial and error. Many servo and proportional valves are manufactured with a spool and sleeve (sometimes called a bushing) as a matched pair. The sleeve has rectangular metering slots placed at precise locations around its periphery and along its length within the manufacturer’s process tolerances. The spool is first machined to fit snugly within its mating sleeve, then its square-cut lands are machined to provide a slight, but excessive amount of physical and apparent overlap. After spool-sleeve fitting, the spool-sleeve assembly is mounted in a functioning hydraulic test fixture from which a complete metering curve can be generated on automatic graphing equipment.
From the graph, a technician estimates the amount of excess physical overlap, removes the spool from the sleeve, places it into a grinding fixture, and has tiny amounts of material removed from the overlapped lands in order to approach the critical lap condition. Next, the spool-sleeve assembly is again tested hydraulically, and this process is repeated until the flow metering curve passes through zero within allowable limits.
This somewhat repetitive process is called flow grinding, and only by judicious estimations is the final metering curve at the optimal condition. However, perfect passage through zero is not necessary. It only needs to be close. Needless to say, flow grinding is costly because it is time consuming and requires skilled technicians.
Click here to read Part 2 of this continuing discussion.