A basic hose assembly consists of fittings, a tube, reinforcement, and a cover. The reinforcement must provide enough support to keep the tube intact and support all the forces created by the pressurized fluid. The tube is a leak-free barrier that contains the fluid and is supported by the reinforcement. The cover serves the same purpose as our skin — to hold everything inside and provide protection from the ambient environment.
As an engineer new to the industry, I accepted the conventional approach to hose reinforcement design. Obviously, the approach has worked well. There was no reason to question the analysis. Then a concern came up. What would happen if we used a different tube wall thickness? The mean diameter of the reinforcement would be different, but the hydrostatic forces and the fitting’s nipple diameter would be the same. To a seasoned hydraulics guy, this didn’t make sense. What is going on? Maybe hose strength should be based on the nipple diameter instead of the mean diameter of the reinforcement.
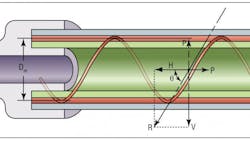
Evans hose reinforcement analysis
Colin Evans’ book, Hose Technology, is a respected engineering resource found in many hose manufacturing engineering libraries. Similar reinforcement engineering design approaches are repeated in several other resources. In Chapter 7, Evans presents a free body diagram, along with an analysis of the forces for the creation of reliable hose designs and manufactured product. Figure 1 is an enhanced rendition of Evans’ free body diagram model showing the forces H and V as acting directly on each braid strand at diameter, Dm.
Evans defines an axial and a radial force and designates them as vectors H and V:
H = P x Dm2 × π ÷ 4, and
V = P × Dm × L ÷ 2.
Where:
H is the horizontal (axial) force vector from fluid pressure,
P is fluid pressure (not burst pressure, Pb),
Dmis mean diameter of the reinforcement,
V is the vertical (radial) force vector from fluid pressure, and
Lis the pitch (lead length) of the spiral.
Because these are vectors, we can calculate the magnitude and direction of the resultant vector, R.
The Evans analysis defines forces based on the reinforcement geometry. This analysis has the virtue of calculating a braid construction that has been used for many years and has been used to develop a large body of hose length designs.
Evans concludes with the (hoop force) burst formula for braided or spiral hose:
Pb = (2 × N × R × sinϴ) ÷ (Dm × L)
Where:
Nis the number of reinforcement ends,
Pb is hydrostatic pressure at burst. This value is measured and verified by experiment,
R is the breaking (tensile) strength of the ligament, and
ϴ is the braid angle: arctan [ 2L÷ (π Dm ) ].
Questions raised
Here is the fly in the ointment: The horizontal force calculation is based on the mean diameter of the reinforcement. So what? Well, consider we have a hose tube with a nominal (tube) inside diameter of 0.25 in., and further assume a pressure of 1000 psi. If we choose a tube wall thickness of 0.030 in. or 0.050 in., how much reinforcement do we need to keep the tube from failing? Assume the pitch is such that the reinforcement angle is 54° 44´and further assume the hydrostatic pressure acts at the mean diameter of the reinforcement.
Evans’ solution allows us to estimate both the burst value and the force the reinforcement must support; that value is N × R. This is true for any pressure from zero to burst pressure. Why is that? First, we’ll rearrange the Evans solution:
P × Dm × L ÷ (2 sin ϴ) = N × R
Now we find that the product of N and R for the tube with the 0.030-in. thick wall is about 130.7 lb. For the tube with the 0.050-in. wall, the product is about 166.6 lb. Why should the thicker, stronger tube need stronger reinforcement? To find out, let’s take a closer look at the free body diagram of a hose assembly that includes the end fitting.
Response to hydrostatic pressure
First, we’ll examine the horizontal force created by the fluid pressure. The magnitude is calculated the same as before, but with one difference. The important diameter is not the braid mean diameter, Dm, but, rather, the end fitting nipple’s outside diameter, Dn. The horizontal force is developed from the pressure acting directly on the nipple; the braid must support the horizontal force (and the vertical force).
The horizontal pressure force, H, always pushes directly against the fitting’s nipple. If the reinforcement wasn’t secured to the connector’s shell or socket, the pressure would push the nipple out of the hose. The horizontal force’s magnitude is the same, regardless of tube’s wall thickness does.
This is where the calculation of the reinforcement requirement changes from that suggested by the Evans model. The magnitude of the reaction force carried by the reinforcement diameter does not change even if the tube wall thickness does. The reaction force is related to the nipple’s outside diameter, and it is carried by the reinforcement!
Now let’s look at the vertical force, V, created by the fluid pressure. Similar to the Evans analysis, we show the vertical force acting at the inside diameter of the tube; it does not act directly on the reinforcement. The reinforcement provides the reaction force to the pressure driven forces applied to the inner surface of the tube.
Justification
The mechanics to justify moving the vertical (radial) force, V, to the inside diameter of the tube is two fold. First, the tube is trapped between the fluid and the reinforcement. The fluid pushes radially outward, and the reinforcement pushes back with equal force. Second, the lay of the reinforcement moves to the neutral angle.
The Evans solution for the direction of the neutral angle as 54° 44´ has been verified by decades of experience. Experience also tells us that the braid will move toward the neutral angle unless it is somehow blocked.
This is because if the horizontal force is greater than the vertical force, the hose reinforcement will elongate until the horizontal and vertical forces are equal. That only happens when the reinforcement braid angle is 54° 44´. The reverse is also true: if the horizontal force is less than the vertical force, the hose reinforcement will shrink until it reaches the neutral angle. This is why hose swells and shortens when pressurized.
To explain, we know from experience that when under pressure, the reinforcement always aligns at the neutral angle. So where does the vertical force act? It must be adjacent to the horizontal force so that the resulting force created by the vector sum of the horizontal and vertical forces lies at the neutral angle.
A suggested improvement
My suggestion is for hose manufacturers to change from using the mean diameter of the reinforcement, Dm, to the connector’s nipple diameter, Dn. Any geometric change to the tube or braid thickness has no impact on the magnitude of the force the reinforcement must support.
In summary, the hydrostatic forces, H and V, act on the inner surface of the tube; that is, at the nipple diameter and along the related wetted pitch inside tube. The resultant force acts along the neutral angle and the magnitude of the resultant force is:
FR = P × 1.36 × Dn2.
Following Evans’ analysis, we can conclude that:
R × N = P × 1.36 × Dn2.
We now have created a model in which we know that the optimum design strength of the reinforcement must be equal to the connector’s nipple diameter instead of the mean diameter of the reinforcement. This means the Evans approach is too conservative for OEMs and users that want a lighter weight, yet equally strong and reliable hose assembly that is designed using the lower forces based on the nipple OD. Adopting this suggested approach may lead to the next generation hose design.
Design theory for optimization
We calculate the hydrostatic force based on the nipple’s diameter, which reduces the hose reinforcement design to N × R. We know the braid angle, so the designer simply selects the solution for N × R.
The minimum number of reinforcement ends, N, must provide sufficient coverage of the outer surface of the hose tube to prevent it from extruding between the reinforcement strands. Furthermore, the product of N and R must exceed the minimum burst pressure. This means that the geometric solution for N × R may require two or more layers of reinforcement to keep the hose tube from failing.
Conclusions
The Evans analysis is successful because it is conservative. It is based on using the mean reinforcement (braid or spiral) diameter as the boundary for generating the hydrostatic force. However, the free-body-diagram at the wetted surfaces indicates the horizontal force is generated by the pressure acting directly on the nipple. The result is that the magnitude of the horizontal and vertical forces is reduced from that calculated by using the mean reinforcement diameter. These reductions will allow manufacturers to design hose that may be lighter and more flexible than hose currently based on the Evans analysis model.
Peter Stroempl is retired after working 33 years in the hydraulics industry.